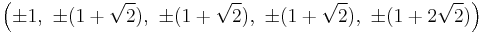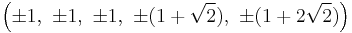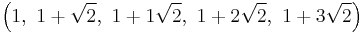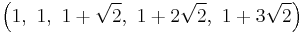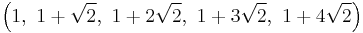Stericated 5-cube
5-cube |
Stericated 5-cube |
Steritruncated 5-cube |
Stericantellated 5-cube |
Steritruncated 5-orthoplex |
Stericantitruncated 5-cube |
Steriruncitruncated 5-cube |
Stericantitruncated 5-orthoplex |
Omnitruncated 5-cube |
| Orthogonal projections in BC5 Coxeter plane | ||
|---|---|---|
In five-dimensional geometry, a stericated 5-cube is a convex uniform 5-polytope with fourth-order truncations (sterication) of the regular 5-cube.
There are eight degrees of sterication for the 5-cube, including permutations of runcination, cantellation, and truncation. The simple stericated 5-cube is also called an expanded 5-cube, with the first and last nodes ringed, for being constructible by an expansion operation applied to the regular 5-cube. The highest form, the steriruncicantitruncated 5-cube, is more simply called a omnitruncated 5-cube with all of the nodes ringed.
Contents |
Stericated 5-cube
| Stericated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,4{4,3,3,3} | |
| Coxeter-Dynkin diagram | ||
| 4-faces | 242 | |
| Cells | 800 | |
| Faces | 1040 | |
| Edges | 640 | |
| Vertices | 160 | |
| Vertex figure | ||
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex | |
Alternate names
- Stericated penteract / Stericated 5-orthoplex / Stericated pentacross
- Expanded penteract / Expanded 5-orthoplex / Expanded pentacross
- Small cellated penteract (Acronym: scan) (Jonathan Bowers)[1]
Coordinates
The Cartesian coordinates of the vertices of a stericated 5-cube having edge length 2 are all permutations of:
Images
The stericated 5-cube is constructed by a sterication operation applied to the 5-cube.
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Steritruncated 5-cube
| Steritruncated 5-cube | |
|---|---|
| Type | uniform polyteron |
| Schläfli symbol | t0,1,4{4,3,3,3} |
| Coxeter-Dynkin diagrams | |
| 4-faces | 242 |
| Cells | 1520 |
| Faces | 2880 |
| Edges | 2240 |
| Vertices | 640 |
| Vertex figure | |
| Coxeter groups | BC5, [3,3,3,4] |
| Properties | convex |
Alternate names
- Steritruncated penteract
- Prismatotruncated penteract (Acronym: capt) (Jonathan Bowers)[2]
Construction and coordinates
The Cartesian coordinates of the vertices of a truncated 5-cube having edge length 2 are all permutations of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Stericantellated 5-cube
| Stericantellated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,2,4{4,3,3,3} | |
| Coxeter-Dynkin diagram | ||
| 4-faces | 242 | |
| Cells | 2080 | |
| Faces | 4720 | |
| Edges | 3840 | |
| Vertices | 960 | |
| Vertex figure | ||
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex | |
Alternate names
- Stericantellated penteract
- Stericantellated 5-orthoplex, stericantellated pentacross
- Cellirhombated penteractitriacontiditeron (Acronym: carnit) (Jonathan Bowers)[3]
Coordinates
The Cartesian coordinates of the vertices of a stericantellated 5-cube having edge length 2 are all permutations of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Stericantitruncated 5-cube
| Stericantitruncated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,1,2,4{4,3,3,3} | |
| Coxeter-Dynkin diagram |
||
| 4-faces | 242 | |
| Cells | 2320 | |
| Faces | 5920 | |
| Edges | 5760 | |
| Vertices | 1920 | |
| Vertex figure | ||
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex, isogonal | |
Alternate names
- Stericantitruncated penteract
- Steriruncicantellated 16-cell / Biruncicantitruncated pentacross
- Celligreatorhombated penteract (cogrin) (Jonathan Bowers)[4]
Coordinates
The Cartesian coordinates of the vertices of an stericantitruncated tesseract having an edge length of 2 are given by all permutations of coordinates and sign of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Steriruncitruncated 5-cube
| Steriruncitruncated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,1,3,4{4,3,3,3} | |
| Coxeter-Dynkin diagram |
||
| 4-faces | 242 | |
| Cells | 2160 | |
| Faces | 5760 | |
| Edges | 5760 | |
| Vertices | 1920 | |
| Vertex figure | ||
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex, isogonal | |
Alternate names
- Steriruncitruncated penteract
- Celliprismatotruncated penteractitriacontiditeron (captint) (Jonathan Bowers)[5]
Coordinates
The Cartesian coordinates of the vertices of an steriruncitruncated tesseract having an edge length of 2 are given by all permutations of coordinates and sign of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Steritruncated 5-orthoplex
| Steritruncated 5-orthoplex | |
|---|---|
| Type | uniform polyteron |
| Schläfli symbol | t0,1,4{3,3,3,4} |
| Coxeter-Dynkin diagrams | |
| 4-faces | 242 |
| Cells | 1600 |
| Faces | 2960 |
| Edges | 2240 |
| Vertices | 640 |
| Vertex figure | |
| Coxeter group | BC5, [3,3,3,4] |
| Properties | convex |
Alternate names
- Steritruncated pentacross
- Celliprismated penteract (Acronym: cappin) (Jonathan Bowers)[6]
Coordinates
Cartesian coordinates for the vertices of a Steritruncated 5-orthoplex, centered at the origin, are all permutations of
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Stericantitruncated 5-orthoplex
| Stericantitruncated 5-orthoplex | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,1,2,4{4,3,3,3} | |
| Coxeter-Dynkin diagram |
||
| 4-faces | 242 | |
| Cells | 2400 | |
| Faces | 6000 | |
| Edges | 5760 | |
| Vertices | 1920 | |
| Vertex figure | ||
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex, isogonal | |
Alternate names
- Stericantitruncated pentacross
- Celligreatorhombated pentacross (cogart) (Jonathan Bowers)[7]
Coordinates
The Cartesian coordinates of the vertices of an stericantitruncated 5-orthoplex having an edge length of 2 are given by all permutations of coordinates and sign of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Omnitruncated 5-cube
| Omnitruncated 5-cube | ||
| Type | Uniform 5-polytope | |
| Schläfli symbol | t0,1,2,3,4{4,3,3,3} | |
| Coxeter-Dynkin diagram |
||
| 4-faces | 242 | |
| Cells | 2640 | |
| Faces | 8160 | |
| Edges | 9600 | |
| Vertices | 3840 | |
| Vertex figure | irr. {3,3,3} |
|
| Coxeter group | BC5 [4,3,3,3] | |
| Properties | convex, isogonal | |
Alternate names
- Steriruncicantitruncated 5-cube (Full expansion of omnitruncation for 5-polytopes by Johnson)
- Omnitruncated penteract
- Omnitruncated 16-cell / omnitruncated pentacross
- Great cellated penteractitriacontiditeron (Jonathan Bowers)[8]
Coordinates
The Cartesian coordinates of the vertices of an omnitruncated tesseract having an edge length of 2 are given by all permutations of coordinates and sign of:
Images
| Coxeter plane | B5 | B4 / D5 | B3 / D4 / A2 |
|---|---|---|---|
| Graph | |||
| Dihedral symmetry | [10] | [8] | [6] |
| Coxeter plane | B2 | A3 | |
| Graph | |||
| Dihedral symmetry | [4] | [4] |
Related polytopes
This polytope is one of 31 uniform polytera generated from the regular 5-cube or 5-orthoplex.
Notes
References
- H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, editied by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6 [1]
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I, [Math. Zeit. 46 (1940) 380-407, MR 2,10]
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II, [Math. Zeit. 188 (1985) 559-591]
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D.
- Richard Klitzing, 5D, uniform polytopes (polytera) x3o3o3o4x - scan, x3o3o3x4x - capt, x3o3x3o4x - carnit, x3o3x3x4x - cogrin, x3x3o3x4x - captint, x3x3x3x4x - gacnet, x3x3x3o4x - cogart
External links
- Glossary for hyperspace, George Olshevsky.
- Polytopes of Various Dimensions, Jonathan Bowers
- Multi-dimensional Glossary